تحويلات أفقية-استوائية

التحويل بين احداثيات أفقية و استوائية

{Note: If your browser does not distinguish between "a,b" and "α, β" (the Greek letters "alpha, beta") then I am afraid you will not be able to make much sense of the equations on this page.}

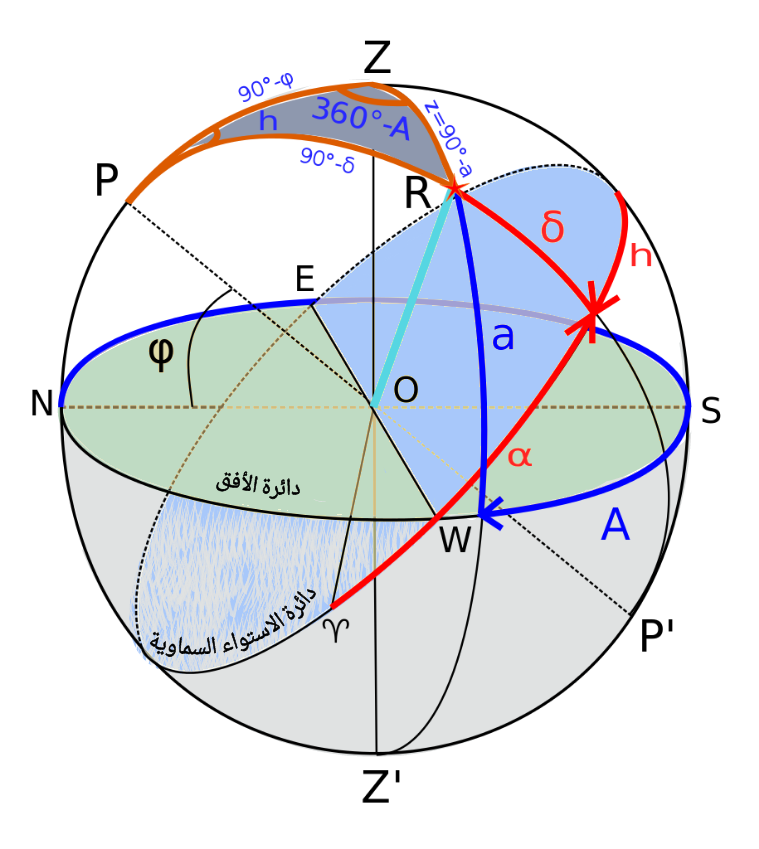
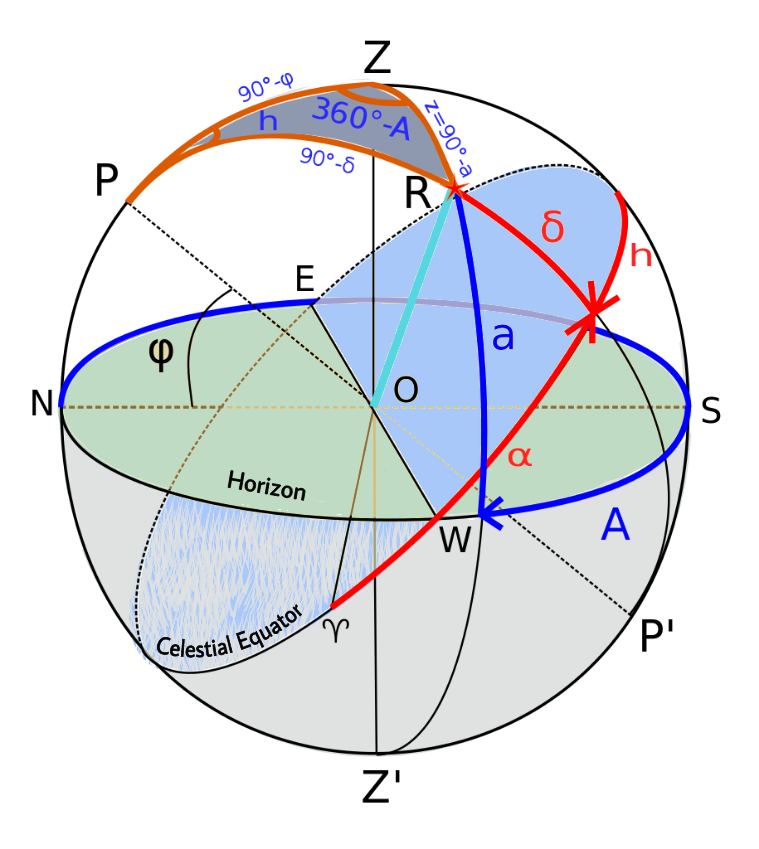
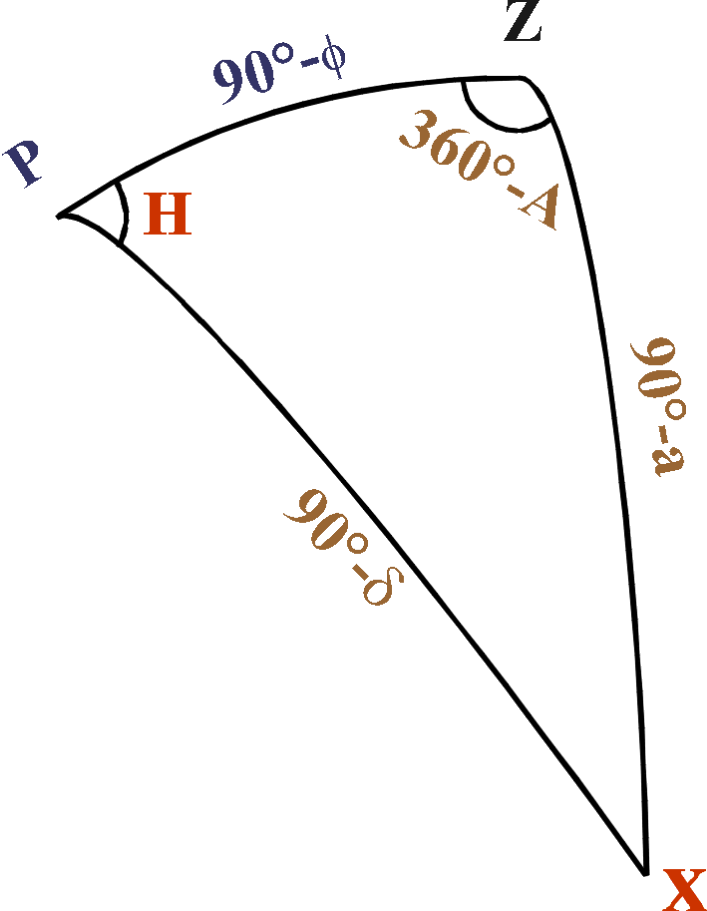
To convert between the horizontal and equatorial coordinates for an object X, we use a spherical triangle often called "The" Astronomical Triangle: XPZ, where Z is the zenith, P is the North Celestial Pole, and X is the object.

The sides of the triangle:
PZ is the observer's co-latitude = 90°-φ.
ZX is the zenith distance of X = 90°-a.
PX is the North Polar Distance of X = 90°-δ.

The angles of the triangle:
   The angle at P is H, the local Hour Angle of X.
   The angle at Z is 360°-A, where A is the azimuth of X.
   The angle at X is q, the parallactic angle.

We assume we know the observer’s latitude φ and the Local Sidereal Time LST.
(LST may be obtained, if necessary, from Greenwich Sidereal Time and observer’s longitude.)

To convert from equatorial to horizontal coordinates:

Given RA α and declination δ, we have
Local Hour Angle H = LST - RA, in hours;
convert H to degrees (multiply by 15).
Given H and δ, we require azimuth A and altitude a.

By the cosine rule:
cos(90°-a) = cos(90°-δ) cos(90°-φ) + sin(90°-δ) sin(90°-φ) cos(H)
which simplifies to:
sin(a) = sin(δ) sin(φ) + cos(δ) cos(φ) cos(H)
This gives us the altitude a.

By the sine rule:
sin(360°-A)/sin(90°-δ) = sin(H)/sin(90°-a)
which simplifies to:
- sin(A)/cos(δ) = sin(H)/cos(a)
i.e. sin(A) = - sin(H) cos(δ) / cos(a)
which gives us the azimuth A.

Alternatively, use the cosine rule again:
cos(90°-δ) = cos(90°-φ) cos(90°-a) + sin(90°-φ) sin(90°-a) cos(360°-A)
which simplifies to
sin(δ) = sin(φ) sin(a) + cos(φ) cos(a) cos(A)
Rearrange to find A:
cos(A) = { sin(δ) - sin(φ) sin(a) } / cos(φ) cos(a)
which again gives us the azimuth A.

Here are all the equations together:

H = t - α

sin(a) = sin(δ) sin(φ) + cos(δ) cos(φ) cos(H)

sin(A) = - sin(H) cos(δ) / cos(a)

cos(A) = { sin(δ) - sin(φ) sin(a) } / cos(φ) cos(a)

Now for the inverse problem: to convert from horizontal to equatorial coordinates:

Given φ, a and A, what are α and δ?

Start by using the cosine rule to get δ, as shown above:
sin(δ) = sin(a)sin(φ) + cos(a) cos(φ) cos(A)

We can now use the sine rule to get H, using the same formula as above:
sin(H) = - sin(A) cos(a) / cos(δ)
Or use the cosine rule instead:
sin(a) = sin(δ)sin(φ) + cos(δ) cos(φ) cos(H)
and rearrange to find H:
cos(H) = { sin(a) - sin(δ) sin(φ) } / cos(δ) cos(φ)

Having calculated H, ascertain the Local Sidereal Time t.
Then the R.A. follows from
α = t – H

Here are all the equations together:

sin(δ) = sin(a)sin(φ) + cos(a) cos(φ) cos(A)

sin(H) = - sin(A) cos(a) / cos(δ)

cos(H) = { sin(a) - sin(δ) sin(φ)} / cos(δ) cos(φ)

α = t – H

تمرين:

Prove that the celestial equator cuts the horizon at azimuth 90° and 270°,
at any latitude (except at the North and South Poles).

At what angle does the celestial equator cut the horizon, at latitude φ ?

الحل:

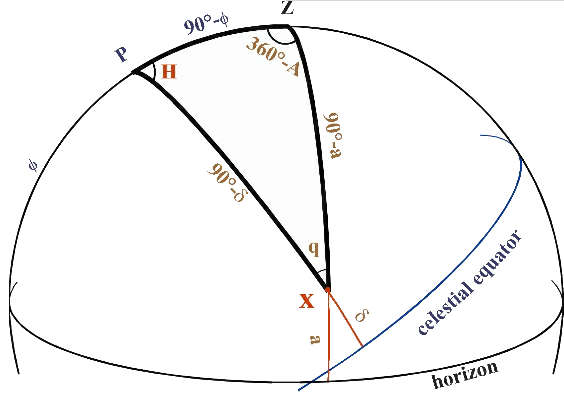
Draw "the" triangle again.
We require the azimuth A of point X,
where X is on the horizon (i.e. a=0)
and also on the equator (i.e. δ=0)

Apply the cosine rule:
cos PX = cos PZ cos XZ + sin PZ sin XZ cos Z
to get 0 = 0 + sin (90-φ) cos A
Since 90°-φ is not 0 (we are not at the Poles),
cos A must be 0
so A = 90° or 270° .

At what angle does the celestial equator cut the horizon, at latitude φ ?

Use the cosine formula:
cos SY = cos SW cos YW + sin SW sin YW cos W
This gives cos (90°-φ) = 0 + cos x
So the angle x is 90°-φ.
The celestial equator cuts the horizon at an angle of 90°-φ

"الفلك الموضعي"

تحويلات أفقية-استوائية

التحويل بين احداثيات أفقية و استوائية

تمرين:

الحل:

ترجمة قتيبة أقرع